The exploration of strongly interacting matter under extreme conditions is one of the most exciting research programs of modern nuclear physics. The recent discoveries of neutron star mergers and supermassive neutron stars challenge our knowledge on high-density QCD matter, like its equation-of- state (EOS) and the microscopic degrees-of-freedom. The mission of the CBM experiment is to produce and investigate dense nuclear matter in energetic heavy-ion collisions, to explore the high-density EOS, and to search for new phases of matter, which may feature characteristic structures such as a first-order phase transition with a region of phase coexistence and a critical endpoint.
Mapping the phase diagram of QCD matter
The conjectured phases of nuclear matter and their boundaries are illustrated in figure 1 in a 3-D diagram of temperature versus baryon-chemical potential μB and isospin-chemical potential mI. Normal nuclei exist in the little turquoise area at T = 0 and finite μB and μI. With increasing T and μB, the nucleons are excited into baryonic resonances which subsequently decay into pions and nucleons. This mixture of nucleons, baryonic resonances and mesons is called hadronic matter, which is produced in heavy-ion collisions at SIS18 energies. The fundamental theory of strong interaction, Quantum Chromo Dynamics (QCD), predicts a crossover chiral phase transition for very low baryon-chemical potentials at a pseudo-critical temperature of about 155 MeV, which is more than hundred-thousand times hotter than the core of the sun. Almost the same value is extracted for the freeze-out temperature from particle yields measured in heavy-ion collision experiments at LHC and top RHIC energies. Above this temperature, QCD matter is expected to dissolve into its constituents, quarks and gluons. A similar mixture of elementary matter and antimatter existed as primordial soup in early universe, about a microsecond after the big bang.
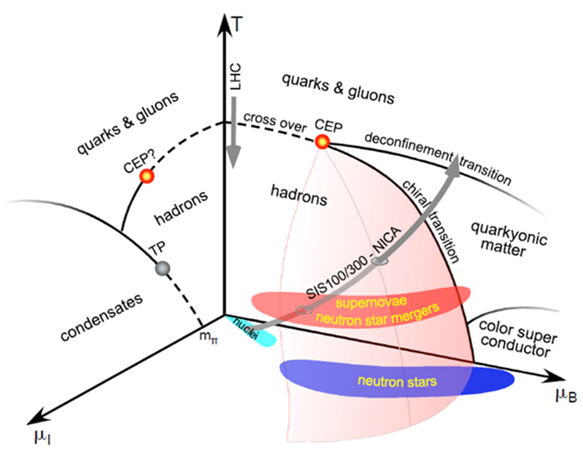
Fig. 1: Sketch of the phase diagram for nuclear matter as function of temperature T, baryon-chemical potential μB, and isospin-chemical potential μI (taken from the NuPECC Long Range Plan 2017 "Perspectives for Nuclear Physics").
The region of the QCD phase diagram at large baryon chemical potential is essentially unknown. Lattice QCD calculations are still limited to low μB, and leave room for effective models, which predict, for example, a first-order phase transition with a critical endpoint. Moreover, exotic phases of QCD matter have been proposed, such as quarkyonic matter, which can be considered as a Fermi gas of free quarks, with all thermal excitations permanently confined. None of these structures and phases have been found so far. In addition to these conjectured landmarks, there should be regions of cosmic matter created in supernovae, neutron stars and neutron star mergers, which are located at large baryon-chemical potentials, low temperatures, and finite isospin chemical potentials, as indicated in figure1. Heavy-ion collisions at FAIR energies permit the exploration of the "terra incognita" of the QCD phase diagram in the region of high baryon densities. This research program is complementary to the investigations performed at RHIC and LHC.
Exploring cosmic matter in the laboratory
An example for cosmic matter is illustrated in figure 2, which depicts the evolution of the temperatures, densities and degrees-of freedom of matter in the collision zone of two neutron stars, as calculated by a model. The left part of the three snapshots displays the temperature T, while the right part presents the quark fraction Yquark. The green lines represent contours of constant baryon density in units of the nuclear saturation density ρ0. The calculation predicts a phase transition to pure quark matter at a density of 4 ρ0 and at a temperature of about 50 MeV. This phase transition occurs shortly before the 2.8 solar mass neutron star collapses into a black hole.
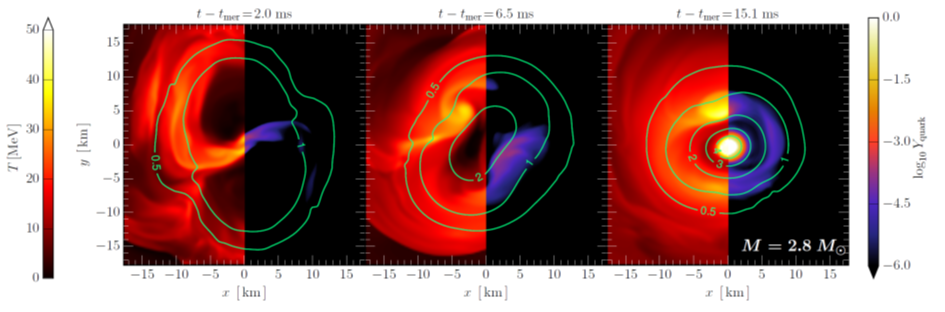
Fig.2: Snapshots on the equatorial plane at three representative times of the evolution of two merging neutron stars with a total mass of 2.8 solar masses. The left and the right part of each plot indicates the temperature and the log10 of the quark fraction Yquark, respectively. The green lines represent contours of constant net- baryon density in units of the nuclear saturation density ρ0. In this model calculation, a phase transition takes place only shortly before the high-mass neutron star collapses to a black hole (see right panel). Taken from E. Most et al., Phys. Rev. Lett. 122 (2019) 061101.
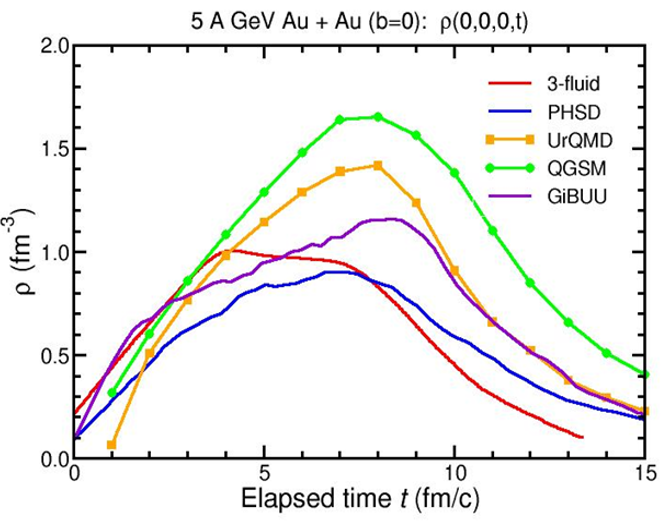
Figure 3: Net-baryon density versus time reached in a central Au+Au collision at 5 A GeV beam energy according to various transport codes and a hydro-dynamical calculation. A net baryon density of ρ=1 fm-3 corresponds to about 7 times the density of an atomic nucleus.
Fundamental questions and experimental observables
The scientific goal of the CBM experiment is to find answers to the following fundamental questions:
- What is the high-density equation-of-state of nuclear matter, which is relevant for our understanding of supernova, the structure of neutron stars, and the dynamics of neutron star mergers?
- What are the relevant degrees of freedom at high densities? Is there a phase transition from hadronic to quark-gluon matter, a region of phase coexistence, and a critical point? Do exotic QCD phases like quarkyonic matter exist? Can we find experimental evidence for the restoration of chiral symmetry, in order to shed light on the generation of hadron masses?
- How far can we extend the chart of nuclei towards the third (strange) dimension by producing single and double hypernuclei? Which role do hyperons play in the core of neutron stars?
These questions will be addressed by measuring the following observables:
- The equation-of-state can be studied by measuring (i) the collective flow of identified particles, which is generated by the density gradient of the early fireball, and (ii) by multi-strange hyperons, which are preferentially produced in the dense phase of the fireball via sequential collisions.
- The existence of a phase transition from hadronic to partonic matter is expected to be reflected in the following observables: (i) the excitation function of multi-strange hyperons, which are driven into equilibrium at the phase boundary; (ii) the excitation function of the invariant mass spectra of lepton pairs which reflect the fireball temperature, and, hence, may reveal a caloric curve and a first-order phase transition; (iii) the excitation function of higher-order event-by-event fluctuations of conserved quantities such as strangeness, charge, and baryon number may be are expected to occur in the vicinity of the critical point (“critical opalescence”).
- Modifications of hadron properties in dense baryonic matter and the onset of chiral symmetry restoration will affect the invariant-mass spectra of di-leptons, which will be measured both in the electron and the muon channel with unprecedented precision.
- The discovery of new (double-Λ) hypernuclei, and the measurement of their life time will provide information on the hyperon-nucleon and hyperon-hyperon interaction, which will shed light on the hyperon puzzle in neutron stars.
An exhaustive review of the physics of hot and dense QCD matter is presented in the CBM Physics Book (link). A more recent overview on the CBM research program can be found in T. Ablyazimov et al., (CBM Collaboration) Eur. Phys. J. A 53 (2017) 60.


